Critical Point of Water
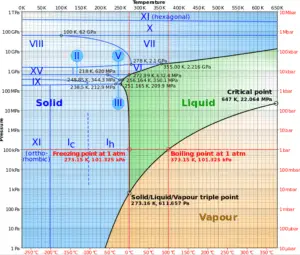
In thermodynamics, a critical point (or critical state) is the end point of a phase equilibrium curve. The phase diagram of water is a pressure-temperature diagram for water that shows how all three phases (solid, liquid, and vapor) may coexist together in thermal equilibrium. Along the vaporization line, the liquid and vapor phases are in equilibrium, along the fusion line, the solid and liquid phases are in equilibrium and along the sublimation line, the solid and vapor phases are in equilibrium. The only point at which all three phases may exist in equilibrium is the triple point. The vaporization line ends at the critical point because there is no distinct change from the liquid phase to the vapor phase above the critical point.
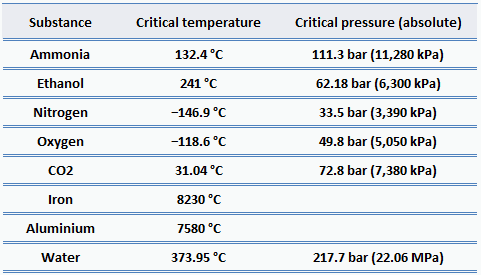
Above the critical point, there is no constant-temperature vaporization process. At the critical point the saturated-liquid and saturated-vapor states are identical. The temperature, pressure, and specific volume at the critical point are called the critical temperature, critical pressure, and critical volume. For water, these parameters are the following:
- Pcr = 22.09 MPa
- Tcr = 374.14 °C (or 647.3 K)
- vcr = 0.003155 m3/kg
- uf = ug = 2014 kJ/kg
- hf = hg = 2084 kJ/kg
- sf = sg =4.406 kJ/kg K
A supercritical phase (e.g. water at a pressure above the critical pressure) does not separate into two phases when it is cooled at constant pressure (along a horizontal line above the critical point in phase diagram). Instead, its properties change gradually and continuously from those we ordinarily associate with a gas (low density, large compressibility) to those of a liquid (high density, small compressibility) without a phase change.
As can be seen from steam tables, as we approach the critical point, the differences in physical properties (such as density and enthalpy) between the liquid and vapor phases become smaller. For example, just below the critical point at the pressure of 21.8 MPa and the temperature of 373°C the specific enthalpy of saturated liquid is 1970 kJ/kg, while the specific enthalpy of saturated vapor is 2230 kJ/kg. The specific heat of vaporization is only 260 kJ/kg. Exactly at the critical point these differences become zero, and at this point the distinction between liquid and vapor disappears. The heat of vaporization also becomes zero at the critical point.
Near the critical point, the physical properties of the liquid and the vapor change dramatically. For example, liquid water under normal conditions has a low thermal expansion coefficient, is nearly incompressible, is an excellent solvent for electrolytes, and has a high dielectric constant. But near the critical point, all these properties change into the exact opposite: water becomes compressible, has a significant thermal expansion coefficient, has a low dielectric constant, is a bad solvent for electrolytes. At pressures greater than the critical pressure the physical properties also undergo a fast transition, but without singularities. With further increase in pressure the transition becomes smoother. This transition region is called pseudocritical region, at a given pressure the temperature where the specific heat has its maximum is called pseudocritical point.
For nearly all familiar materials the critical pressures are much greater than atmospheric pressure, therefore we don’t observe this behavior in everyday life. But in energy engineering, there are many applications for supercritical water. One of ways to increase thermal efficiency of thermodynamic cycles (e.g. Rankine cycle) is to increase the peak temperature and pressure in the boiler. High-pressure steam boilers in thermal power plants regularly run at pressures and temperatures well above the critical point.
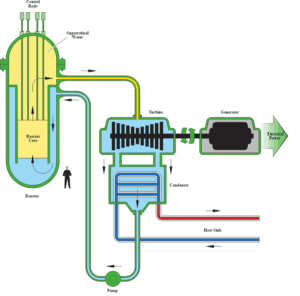
Author (Public domain): U.S. Department of Energy
In nuclear engineering, the supercritical water reactor is considered a promising advancement for nuclear power plants because of its high thermal efficiency (~45 % vs. ~33 % for current LWRs). This concept of reactor operates at supercritical pressure (i.e. greater than 22.1 MPa) and belongs to Generation IV reactor designs.
See also: Supercritical Water Reactor
We hope, this article, Critical Point of Water, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about thermal engineering.